A Graphene Drum
September 22, 2014
The
cantilever, as shown in the figure, is a simple
mechanical object whose properties are defined by its dimensions and the
properties of the material from which it is made. Cantilevers can be used as the basis of a variety of devices, as I've written in some previous articles (
Weighing Attograms, January 27, 2014;
Energy Harvesting Cantilevers, January 14, 2013;
Combinatorial Research, December 12, 2011;
Cantilever Energy Harvesting, August 16, 2011; and
Paper Accelerometer, March 10, 2011.

The mechanical properties of a cantilever of uniform cross-section are defined by its length L, width w, and thickness t, along with the material properties of Young's modulus E, and density ρ.
(Illustration by the author, rendered using Inkscape.)
A cantilever will "ring" at a characteristic
resonant frequency,
fr, when you pluck it,
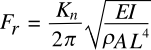
where
Kn is a constant that specifies the resonant mode (3.516 for the first mode),
E is
Young's modulus,
I is the
moment of inertia,
A is the cross-sectional area (
w⋅t),
ρ is the
density, and
L is the length. The moment of inertia of a cantilever is easy to
calculate, but it's even easier to just list,
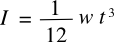
Combining the
equations gives the following result,

What's interesting about this is that the resonance frequency is
independent of the width of the cantilever beam. More importantly, the density term means that the frequency will change with
mass loading. It will, of course, depend on where the mass is added, but the change will be
in proportion to m
-1/2.
The mass response of the cantilever resonant frequency became important with the advent of
microelectromechanical systems (MEMS), since it was then possible to make
silicon cantilever resonators with
attogram (10
-18 gram) mass
sensitivity. This is about the weight of individual large
protein molecules, so cantilever
immunoassay sensors were possible.
Since mass detection depends on the initial mass of a resonator, just how small can you make a resonator? In 2012,
scientists from the
US National Institute of Standards and Technology (NIST, Gaithersburg, Maryland), and the
University of Maryland (College Park, Maryland) made
drumhead resonators of
graphene by suspending it over shallow holes in a
silicon wafer.[1-2] Although their objective was the modification of graphene's
electrical properties, they found that they could use the
van der Waals forces from the tip of a
scanning tunneling microscope to move the graphene membrane.[1-2]
Now, scientists from the
Kavli Institute of NanoScience at the
Delft University of Technology (Delft, The Netherlands) have created graphene drumheads over
superconducting cavities and used
microwave photons to both tap these drumheads and "listen" to their response.[3-7] Says
Vibhor Singh, first
author of the
paper describing this
research and a scientist at the Kavli Institute of NanoScience,
"In this experiment, we shot microwave photons at a tiny graphene drum. The drum acts as a mirror: by looking at the interference of the microwave photons bouncing off of the drum, we are able to sense minute changes in the position of the graphene sheet of only 17 femtometers, nearly 1/10000th of the diameter of an atom."[4]
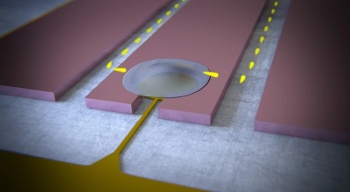
Artist's impression of the graphene drumhead.
(Kavli Institute of Nanoscience, still image from a YouTube video.)
The tapping
force is from the very small
momentum of the microwave photons. I discussed photon momentum in a
previous article (The Pioneer Anomaly, December 19, 2012). Although the photon momentum is small, the mass of the graphene sheet is likewise small, so displacement of the graphene is detectable.[4,6] The graphene resonators had
quality factors up to 220,000 and displacement sensitivity of 17
fm Hz−1/2.[3] The Kavli researchers were able to achieve microwave
amplification with these devices. Seventeen
dB of microwave amplification was achieved.[3] A
YouTube video shows the action of these drumheads.[7]
Since graphene is an atomic layer material, its properties verge on the
quantum mechanical realm, and the Kavli research team thinks that similar devices may be used as
quantum computing elements. Kavli scientist,
Gary Steele, describes the quantum mechanical aspects, as follows:
"If you hit a classical drum with a stick, the drumhead will start oscillating, shaking up and down. With a quantum drum, however, you cannot only make the drumhead move up and then down, but also make it into a 'quantum superposition', in which the drum head is both moving up and moving down at the same time... This 'strange' quantum motion is not only of scientific relevance, but also could have very practical applications in a quantum computer as a quantum 'memory chip'."[5]
References:
- Nikolai N. Klimov, Suyong Jung, Shuze Zhu, Teng Li, C. Alan Wright, Santiago D. Solares, David B. Newell, Nikolai B. Zhitenev, Joseph A. Stroscio, "Electromechanical Properties of Graphene Drumheads," Science, vol. 336, no. 6088 (June 22, 2012), pp. 1557-1561.
- Dexter Johnson, "Tightening Graphene Like a Drumhead Changes Its Electrical Properties," IEEE Spectrum, July 12, 2012.
- V. Singh, S. J. Bosman, B. H. Schneider, Y. M. Blanter, A. Castellanos-Gomez, and G. A. Steele, "Optomechanical coupling between a multilayer graphene mechanical resonator and a superconducting microwave cavity," Nature Nanotechnology, Advanced Online Publication, August 24, 2014, doi:10.1038/nnano.2014.168.
- Delft researchers play the graphene drum, Delft University of Technology Press Release, August 25, 2014. Dutch language press release.
- Tiny graphene drum could form future quantum memory, Delft University of Technology Press Release, August 25, 2014.
- Dexter Johnson, "Graphene Drumheads Could Lead to New Sensors for Mobile Phones," IEEE Spectrum, August 28, 2014
- TU Delft, Resonator Animation, YouTube Video, August 25, 2014
Permanent Link to this article
Linked Keywords: Cantilever; mechanics; mechanical; material properties; Young's modulus; density; Inkscape; resonance; resonant frequency; Young's modulus; moment of inertia; density; calculation; calculate; equation; independent variable; mass; proportionality; microelectromechanical systems; silicon; attogram; sensitivity; protein molecule; immunoassay; sensor; scientist; US National Institute of Standards and Technology (NIST, Gaithersburg, Maryland); University of Maryland (College Park, Maryland); drumhead; resonator; graphene; silicon wafer; electrical properties; van der Waals force; scanning tunneling microscope; Kavli Institute of NanoScience; Delft University of Technology (Delft, The Netherlands); superconductivity; superconducting; microwave; photon; Vibhor Singh; author; scientific literature; paper; research; mirror; interference; femtometer; diameter; atom; artist's impression; YouTube video; force; momentum; quality factor; fm; hertz; Hz; amplifier; amplification; decibel; dB; quantum mechanics; quantum mechanical; quantum computer; quantum computing; component; Gary Steele; quantum superposition; memory chip.