| Tikalon Blog is now in archive mode.
An easily printed and saved version of this article, and a link
to a directory of all articles, can be found below: |
|
This article |
| Directory of all articles |
Coiling
October 20, 2014
In previous articles (
Crumpling, August 27, 2012,
Packing, November 30, 2010 and
Packing and Filling, May 17, 2012), I've discussed
crumpling,
packing and filling, and
stacking. Crumpling involves
two-dimensional objects, such as a sheet of
paper, while packing, filling, and stacking involve three-dimensional objects, such as
granular materials.
Additional to these two- and three-dimensional processes, there' s an important
one-dimensional material process, folding. The most important example of folding is
protein folding.
Proteins are
biological macromolecules formed when a
polypeptide, which is a linear chain of
amino acid residues, folds into a three-dimensional structure. Just as the
isomeric forms of small molecules affect
molecules their
chemical activity, the way that proteins fold affects their biological function. Incorrect protein folding, known as
proteopathy, can cause
disease.
A simplified example of the main driving force behind the folding that leads to the final conformation of a protein molecule.
Hydrophobic amino acids (blue spheres) are better shielded from the solvent environment when folded as shown on the right.
(Modified Wikimedia Commons image)
A minimally functional protein consists of about 40-50 amino acid residues, and some multi-functional proteins will contain several hundred amino acid residues. As first noticed in 1969 by
American molecular biologist,
Cyrus Levinthal, a chain of just a hundred residues would still have a
myriad number ways in which to fold.
The number is too large to allow an
exhaustive search for a lowest
energy conformation, either through the physical motion of the molecules themselves, or by
computer simulation, but proteins are known to fold in just
milliseconds. This contradiction is called
Levinthal's paradox, but it hasn't stopped attempts at
computer-assisted protein structure prediction, such as the
folding@home distributed computing project.
Every home has an assortment of
garden hoses,
extension cords, and
computer cables, all of which we wouldn't want reduced to a three-dimensional tangle reminiscent of a folded protein. We're instead interested in how these might be
coiled. Coiling is the folding process with a dimensional restriction.
Intermediate between proteins and garden hoses are bacterial flagella. Flagella propel bacteria to better food sources. (Still image, enhanced, from an MIT YouTube Video.)
Scientists and
engineers from the
Department of Mechanical Engineering and
Department of Civil and Environmental Engineering of the
Massachusetts Institute of Technology (Cambridge, Massachusetts), and the
Department of Computer Science at
Columbia University (New York, NY), have
experimentally examined how
flexible cables coil on static and moving substrates. The results of their studies have been published in the
Proceedings of the National Academy of Sciences.[2-5]
The coiling of cables may sound like a niche
research area, but it's important to our
Internet-connected age since it relates to the laying of
submarine cables. Identifying which
mechanical properties of such cables affects their deployment would prevent such things as cable
breakage, or laying the cables too loosely. Any
twist or tangle in a cable will cause degradation of the
optical signals. Presently, there are more than 550,000
miles of undersea cables.[4] Says MIT lead researcher,
Pedro Reis,
“If the boat is sailing slower than the rate of the cable, then you're putting more cable down, which generates loops, coils, and tangles... That can lead to signal attenuation. But if the boat is traveling faster, then the cable can get taut and fracture, which is really bad news. So we wanted to understand what was underlying those patterns.”[4]
The MIT
laboratory experiments were done with an equivalent
geometry of having a static source dropping cable onto a
conveyor belt (see photograph). The
apparatus allowed adjustment of the
speed of deployment and the speed of the belt, and it included
video capture for
analysis of the results.[4]
Filaments of
silicone rubber were used, and three main patterns were observed; namely,
meandering waves, alternating loops, and repeated coils.[4]
Apparatus for the coiling experiments.
A thin silicone filament (green) is layed on a black conveyor belt to form a periodic array of loops in alternating directions.
(MIT photo by John Freidah.)[4)]
To make sense of the results, the MIT engineers enlisted the aid of
Eitan Grinspun and his team of
computer scientists at Columbia University. As all
programmers know,
code-reuse is a wonderful thing, and Grinspun has developed
computer algorithms for
simulation of the movement of the thin filaments of
hair and
cloth, notably for the
films, “
The Hobbit” and “
Tangled.” Going beyond mere
visual effects, Grinspun has modeled the flow of
honey. Honey, a
viscous fluid, as poured from a jar, can appear as a rope or thread, forming wavelike patterns on a surface. [4]
The
modeling results were initially disappointing until they added the natural
curvature of the filament as a
parameter. Semi-stiff filaments, when wound on a
spool, retain a short-term memory of the spool's curvature. In the end, the model incorporated the speed that the filament was deployed, the speed of the conveyor belt, the stiffness and diameter of the filament, and the size of its spool.[2,4]
The research team also found that the
height from which a filament is deployed didn't have an affect. Says Grinspun,
“This is important because, as a ship sails, the height of the ocean floor relative to the surface is changing all the time... We also know that how big you make the spools on the ship does matter. So we now have a map of how cables coil, and an understanding of what variables are important if you're trying to achieve certain patterns.”[4]
This research was funded, in part, by the
National Science Foundation.[4]
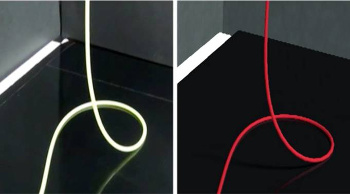
A comparison of experiment (left), and a computer model (right). It was found that the loops form in an alternating clockwise, then counter-clockwise, pattern.
(MIT photograph by the Reis research team.)[4-5)]
References:
- Cyrus Levinthal, "How to Fold Graciously," in Mossbauer Spectroscopy in Biological Systems: Proceedings of a meeting held at Allerton House, Monticello, Illinois, J. T. P. DeBrunner and E. Munck, Eds., University of Illinois Press (1969), pp. 22-24 .
- Mohammad K. Jawed, Fang Da, Jungseock Joo, Eitan Grinspun, and Pedro M. Reis, “Coiling of elastic rods on rigid substrates,” Proc. Natl. Acad. Sci., published ahead of print, September 29, 2014, doi:10.1073/pnas.1409118111.
- Supplemental Information for ref. 2.
- Jennifer Chu, "Untangling how cables coil," MIT Press Release, October 3, 2014.
- Movie: Pattern of alternating loops obtained when depositing filament. See ref. 3 for details.
- The Wonders of Thin Structures from Failure to Functionality, MIT YouTube Video, November 12, 2013.
Permanent Link to this article
Linked Keywords: Crumpling; relative density; packing and filling; sphere packing; stacking; two-dimensional; paper; granular material; one-dimensional; material; protein folding; protein; biology; biological; macromolecule; peptide; polypeptide; amino acid residue; isomer; isomeric form; molecule; chemical reaction; chemical activity; proteopathy; disease; protein structure; conformation; hydrophobe; hydrophobic; amino acid; solvent; Wikimedia Commons; American; molecular biologist; Cyrus Levinthal; myriad; brute-force search; exhaustive search; energy; computer simulation; millisecond; Levinthal's paradox; computer-assisted; protein structure prediction; folding@home; distributed computing; garden hose; extension cord; computer cable; coil; bacteria; bacterial; flagellum; flagella; propulsion; propel; food; YouTube Video; scientist; engineer; Department of Mechanical Engineering; Department of Civil and Environmental Engineering; Massachusetts Institute of Technology (Cambridge, Massachusetts); Department of Computer Science; Columbia University (New York, NY); experiment; experimental; flexible; Proceedings of the National Academy of Sciences; research; Internet; submarine cable; mechanical property; fracture; breakage; twist; optics; optical; signal; mile; Pedro Reis; boat; attenuation; laboratory; geometry; conveyor belt; apparatus; speed; video capture; analysis; fiber; filament; silicone rubber; meandering wave; John Freidah; Eitan Grinspun; computer scientist; programmer; code-reuse; computer algorithm; computer simulation; hair; textile; cloth; film; The Hobbit; Tangled; visual effect; honey; viscous fluid; modeling; curvature; parameter; cable reel; spool; height; ocean floor; National Science Foundation.