Koch Snowflake
November 6, 2023
You Bet Your Life was a popular
game show hosted by
Groucho Marx (1890-1977), first on
radio, then on
television, during the
1940s and
1950s. To ensure that every
contestant would leave with some
prize, Marx would ask a question such as, "Who's
buried in
Grant's tomb?" Grant's tomb is a huge
mausoleum in
New York City containing the remains of
American Civil War General,
Ulysses S. Grant (1822-1885), and his
wife. The accepted answer was
Grant. However, Grant and his wife are not actually below
ground in the mausoleum; so, no one is actually buried in Grant's tomb.[1]
I was reminded of this question when I read a recent
arXiv paper by
Yann Demichel of the
Université Paris Nanterre entitled,
Who invented von Koch's Snowflake Curve?.[2] As Demichel writes, "...the
image of the snowflake
curve is not present or even mentioned in von Koch's original articles."[2] While the 1904 paper, "Sur une courbe continue sans tangente, obtenue par une construction géométrique élémentaire" (On a continuous curve without tangents constructible from elementary geometry),
cited as its origin has several
figures, none of these are the snowflake.[3]
The
mathematical object known as the
Koch snowflake is a
fractal curve credited to
Swedish mathematician,
Helge von Koch (1870-1924). The curve has a
fractal dimension (Hausdorff dimension) of log 4/log 3, about 1.26. The snowflake, as shown in the figure, is simply
constructed, as follows:[4]
• Draw an equilateral triangle.
• Taking each line segment in turn, divide it into three equal segments.
• Draw an equilateral triangle pointing outwards using the middle segment as the base.
• Remove the base.
• Continue this operation for the next two line segments of the original triangle; and then recursively for all line segments.
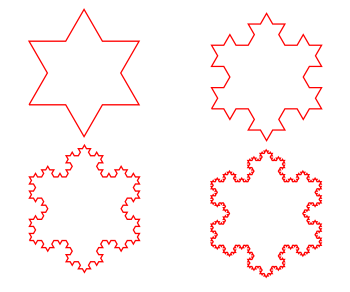
The Koch snowflake in its first-fourth iteration. The starting figure, an equilateral triangle, is omitted.
This fractal is much easier to generate and visualize than the popular Mandelbrot set, which has a Hausdorff dimension of two.
(Rendered using Inkscape from this Python program. Click for larger image.)
As for who was the first to produce the Koch snowflake, Demichel traces its first example to a 1940
popular science book,
Mathematics and Imagination,
co-authored by
Edward Kasner (1878-1955), a
professor at
Columbia University (New York, NY), and one of his
students.[2] The snowflake is shown in an
appendix entitled,
Pathological Curves, but von Koch is not mentioned.[2] Demichel further
speculates that the
snowflake name might have been suggested by a
child in Kasner's
family, and that Kasner could have designed the snowflake curve prior to 1916.[2]
A popular
hobby among
amateur radio operators is making
radio antennas from unusual objects. The first
fractal antenna was
patented in 2002,[5] and there's an
online exposition of a Koch snowflake antenna with interesting
broadband properties[6] (see figure).

Left, figure 7E of US Patent No. 6,452,553, "Fractal antennas and fractal resonators," by Nathan Cohen, September 17, 2002; and, right, a Koch snowflake antenna. Engineers will notice the resemblance of the antenna on the left to a twin-lead "T" antenna. (Left image via Google Patents,[5] and right image (modified) from Ref. 6.[6] Click for larger image.)
I wrote about some interesting
properties of actual
snowflakes in an
older article (Snowflakes, January 18, 2011). The foremost question about snowflakes is whether it's really true that no two snowflakes are alike. At the
atomic level, a snowflake will contain about 10
18 water molecules, some of which might be formed from
deuterium, rather than
hydrogen. On
Earth, there's one deuterium atom for every 6,420 atoms of hydrogen in water. There's no need to
calculate the
permutation, since it's
intuitive that even with the estimated 10
24 snowflakes
falling per
year, the
odds of any two matching is indistinguishable from zero.[7]
Hooke's 1665 book,
Micrographia, included many
drawings of snowflakes, and these were the first drawings to indicate the
complex shapes within shapes in snowflakes. More than two hundred years later, starting in 1885, after the invention of
photography,
Vermont farmer,
Wilson Alwyn Bentley, took numerous
photomicrographs of snowflakes. His photographs documented the fine details inherent in snowflakes and showed
anecdotally how unlikely that two could be the same (see figure).

Snowflakes photographed at Jericho, Vermont, by Wilson Alwyn Bentley, 1902. (Wikimedia Commons images.)
References:
- Quiz Question: Who Is Buried in Grant’s Tomb? Answer: Grant!, Quote Investigator, November 10, 2011.
- Yann Demichel, "Who invented von Koch's Snowflake Curve?" arXiv, August 29, 2023, https://doi.org/10.48550/arXiv.2308.15093.
- Helge von Koch, "Sur une courbe continue sans tangente, obtenue par une construction géométrique élémentaire". Ark. Mat. Astron. Fys., vol. 1 (1904), pp. 681-704.
- Koch Snowflake page on Wikipedia.
- Nathan Cohen, "Fractal antennas and fractal resonators," US Patent No. 6,452,553 , September 17, 2002.
- KB7QHC, "Koch Snowflake Antenna," QSL.net.
- SnowCrystals.com, created by Kenneth G. Libbrecht of Caltech.
Linked Keywords: You Bet Your Life; game show; radio personality; host; Groucho Marx (1890-1977); radio broadcasting; television program; 1940s; 1950s; contestant; prize; burial; buried; Grant's tomb; mausoleum; New York City; American Civil War; General; Ulysses S. Grant (1822-1885); wife; soil; ground; arXiv; academic publishing; paper; Yann Demichel; Université Paris Nanterre; image; curve; citation; cited; geometry; figure; mathematics; mathematical; Koch snowflake; fractal; Swedish; Helge von Koch (1870-1924); fractal dimension; Hausdorff dimension; geometric construction; constructed; equilateral triangle; line segment; base (geometry); recursion; recursive; Koch snowflake in its first-fourth iteration; scientific visualization; visualize; Mandelbrot set; koch.py; Python program; popular; science; book; author; co-author; Edward Kasner (1878-1955); professor; Columbia University (New York, NY); student; addendum; appendix; speculative reason; speculate; child; family; hobby; amateur radio operator; antenna (radio); radio antenna; fractal antenna; patent; patented; Internet; online; electromagnetic spectrum; broadband; US Patent No. 6,452,553, 'Fractal antennas and fractal resonators,' by Nathan Cohen, September 17, 2002; Engineer; twin-lead; Google Patents; physical property; snowflake; atom; atomic; water; molecule; deuterium; hydrogen; Earth; calculation; calculate; permutation; intuition; intuitive; precipitation (meteorology); falling; year; odds; Micrographia; drawing; complexity; complex; geometry; shape; photography; Vermont; Wilson Alwyn Bentley; photomicrograph; anecdotal evidence; anecdotal; Wikimedia Commons image.