| Tikalon Blog is now in archive mode.
An easily printed and saved version of this article, and a link
to a directory of all articles, can be found below: |
|
This article |
| Directory of all articles |
Technological Progress
May 1, 2013
Everyone is concerned with
progress. During my career in
industrial research, I was required to write monthly and annual progress reports on my
research. I always thought that these were just filed away, somewhere, and they were never read. The important
milestone for some
administrator was putting a
check mark after my name on some sheet to signify that a report had been submitted.
Eventually, such reports migrated to a
database application, and from that point I was even more convinced that whatever I typed into the text boxes was never read. I wrote about this in a
previous article (Untouched by Human Hands, January 25, 2011).
I had the occasion to work with a respected
scientist who had
emigrated to our
laboratory from another company (colloquially called "
Big Blue," but best left unnamed). His former employer also required his writing an annual report, and the form for this contained the question, "What did you accomplish last year?" As a true
experimentalist, he decided to test the system; so, he wrote, "Absolutely nothing." He never got any
feedback on that answer.
At some level, progress is important, since it translates into money. Using the old
analogy, if you're still making
buggy whips, your company will lose business to those that progressed into the supply of accessories for newer vehicles. That's why the better companies still have
R&D laboratories.
If buggy-whip manufacturers had better lobbyists.
This photograph is actually from a Finnish horse-pulling contest in 1948.
Photo by Väinö Erälä, via (Wikimedia Commons.)
As individual companies progress, they carry their industry with them. One of the best equalizers, as
Silicon Valley has found (and has been called to task for illegally trying to stop), is the roving employee who quits one company and brings his general experience to another without compromising any
trade secrets. Experience applies to
industrial processes, also, giving rise to
experience curve effects.
In general, every time the cumulative volume of a
good or
service doubles, cost falls by a constant percentage. This cost advantage generally arises from the fact that it takes less time to perform a task after the task has been done many times. One example of this was presented in a 1936 study by T.P. Wright that showed that the required
labor to produce an
aircraft decreased by 10-15 percent when the quantity produced doubled.[1-2]
Another consequence of experience is an improvement in the
quality or
performance of an item.
George Dyson, in his book, "Turing’s Cathedral,"[3] gives the following example from
vacuum tube days. The simplest active device available for
logic operation at that time was the
triode; so, the 6J6, a dual triode in a
miniature glass envelope (see figure), was an obvious choice for early
computers. What was fortunate was the fact that this tube was produced in large quantities during
World War II, and it was found to be more reliable than other tubes especially crafted for high reliability.[4]
Data on the 6J6A miniature dual-triode vacuum tube, an improved version of the 6J6. (Scan from author's copy of the RCA Receiving Tube Manual, Radio Corporation of America, November, 1966).
The best known experience effect is
Moore's Law, conceived by
Intel co-founder,
Gordon E. Moore, in 1965. Moore's original prediction was a doubling of the number of
transistors in the
highest density integrated circuits every year. The law is now showing a two-year doubling period, but it's often been called a
self-fulfilling prophesy, since
integrated circuit manufacturers are using the law as their
technology roadmap. In 1965, there were barely a thousand transistors on a chip; now, there's billions.
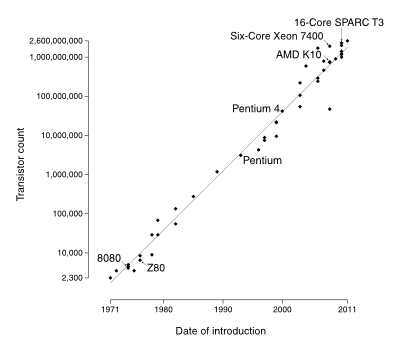
Moore's Law.
The line specifies a two year doubling.
My first personal computers used the 8080 and Z80 microprocessors, which are among the highlighted chips.
(Graph by W.G. Simon (simplified), via Wikimedia Commons.)
Moore's Law applies generally to many industrial processes, with the exponent for the rate of improvement depending on the
technology.[5] The change rate varies greatly over a range of technologies.[5]
So, we have two basic laws that describe technology trends - Wright's Law, that cost decreases as a function of cumulative production; and, Moore's law, that technologies improve exponentially with time (see the figure below for examples). A research team of members from the
Santa Fe Institute,
Oxford University,
St. John's College (Santa Fe, New Mexico) and the
Massachusetts Institute of Technology, has analyzed the compatibility of these two laws in an
open access,
peer-reviewed article in
PLoS One.[5-7]
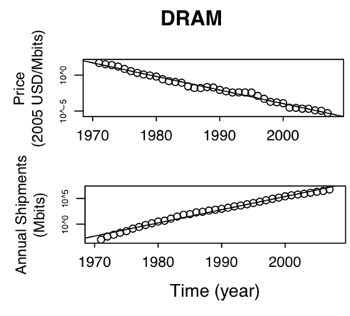
Logarithms of price and production as a function of time for dynamic random-access memory DRAM chips.
(A portion of fig. 3
from ref. 6, licensed under the the Creative Commons Attribution License.)[6)]
As can be expected, such an analysis is a lot of work. The study authors assembled data for cost and production for sixty-two industries, including
beer, the
aluminum from which
beer cans are made, and advanced
electronic products such as computers,
solar cells, aircraft and
automobiles.[5] Source material was only considered relevant when there were at least a decade's worth of data for calculating metrics for both production rate and some measure of improvement.[5]
They used these data to check the validity of industrial
models proposed in the
scientific literature, what they term, "hindcasting," and they found the most valid model to be one proposed by Devendra Sahal in 1979 (see figure).[8] The study authors conclude that this result can aid in "assessments of candidate technologies and policies for climate change mitigation."[PLoS] Says study coauthor,
Jessika Trancik of MIT,
"Information technologies improve the fastest... but you also see the sustained exponential improvement in many energy technologies. Photovoltaics improve very quickly. One of our main interests is in examining the data to gain insight into how we can accelerate the improvement of technology."[5]
The value of the Wright parameter W plotted against the ratio of the exponent of cost reduction and the exponent of the increase in cumulative production, m/g.
(Fig. 4 from ref. 6 , licensed under the the Creative Commons Attribution License.)[6]
This research was funded by the
National Science Foundation, with additional funding provided by
The Boeing Company.[6]
![]()
References:
- T. P. Wright. "Factors Affecting the Cost of Airplanes", Journal of the Aeronautical Sciences (Institute of the Aeronautical Sciences), Vol. 3, No. 4 (1936), pp. 122-128.
- Robert J. Banis, Learning Curves (A summary of Wright's 1936 paper), University of Missouri-St. Louis Web Site.
- George Dyson, "Turing's Cathedral: The Origins of the Digital Universe," Vintage (December 11, 2012), 464 pages. ISBN-13: 978-1400075997 (via Amazon).
- Gwern Branwen, "Slowing Moore's Law: Why You Might Want To and How You Would Do It," March 16, 2012 - 03 April 3, 2013.
- David L. Chandler, "How to predict the progress of technology," MIT Press Release, March 6, 2013.
- Béla Nagy, J. Doyne Farmer, Quan M. Bui and Jessika E. Trancik, "Statistical Basis for Predicting Technological Progress," PLoS ONE, vol. 8, no. 2 (February 28, 2013), Document No. e52669, doi:10.1371/journal.pone.0052669.
- Béla Nagy, J. Doyne Farmer, Jessika E. Trancik and Quan Minh Bui, "Testing Laws of Technological Progress," (An early PDF draft of the above paper), Santa Fe Institute Web Site, September 2, 2010.
- Devendra Sahal, "A theory of progress functions," AIIE Transactions, vol. 11, no. 1 (1979), pp. 23–29.
Permanent Link to this article
Linked Keywords: Idea of Progress; progress; industrial research; research; milestone; business administration; administrator; check mark; database application; scientist; emigrated; laboratory; Big Blue; experimentalist; feedback; analogy; buggy whip; research and development; R&D; lobbying; lobbyist; Finland; Finnish; horse-pulling contest; Wikimedia Commons; Silicon Valley; trade secret; industrial process; experience curve effect; good; service; employment; labor; aircraft; quality; performance; George Dyson; vacuum tube; boolean algebra; logic operation; triode; miniature tube; miniature glass envelope; computer; World War II; RCA; Radio Corporation of America; Moore's Law; Intel; Gordon E. Moore; transistor; very-large-scale integration; highest density integrated circuits; self-fulfilling prophesy; semiconductor device fabrication; integrated circuit manufacturer; technology roadmap; 8080; Z80; microprocessor; technology; Santa Fe Institute; University of Oxford; Oxford University; St. John's College (Santa Fe, New Mexico); Massachusetts Institute of Technology; open-access journal; peer-review; PLoS One; dynamic random-access memory; DRAM; Creative Commons Attribution License; beer; aluminum; beverage can; beer can; electronics; electronic product; solar cell; automobile; computer simulation; model; scientific literature; Jessika Trancik; National Science Foundation; The Boeing Company.