| Tikalon Blog is now in archive mode.
An easily printed and saved version of this article, and a link
to a directory of all articles, can be found below: |
|
This article |
| Directory of all articles |
Snowflakes
January 18, 2011
Today is another
snow day in
Northern New Jersey. We've had more snow this season than our usual share, but that's probably just a
statistical fluctuation that will be balanced by a milder
winter some year in the future. It wouldn't have done us much good to have retreated to the south, since the
US southern states have had an unusually severe winter, also. Being stuck in the house, looking out at all that snow, has me thinking about the physical properties of
ice, which is what snow really is.
About eight inches of snow fell on Northern New Jersey, January 12, 2011 (Photo by author).
Ice is important to the plot of
Kurt Vonnegut's novel,
Cat's Cradle. The ice in this case is
ice-nine, a fictional phase of ice with a name that's similar to the actual ice phase,
ice-IX. Ice-IX is a tetragonal crystalline phase of ice that's stable at very low temperature (< 140
K) and high pressures (200-400
MPa). Ice-IX has a
density of 1.16 g/cm
3. This is quite unlike the ice outside my window, which has a density of about 0.92, so it floats on
water. Fifteen different phases of ice are known, each existing in its own temperature and pressure range. Not surprisingly, the snow outside my window is known as Ice-I. It's even possible to have ice at room temperature and pressure when it's confined to a small dimension.[1-2]
Water and ice have many unusual properties because they're held together by
hydrogen bonds. There are so many unusual properties that there are web sites whose sole purpose is to catalog the
anomalous properties of water; anomalous, that is, compared to other liquids.[3] One interesting property is the huge change in the high frequency
dielectric constant when water freezes. At
MHz frequencies and about 0-20
oC, water has a dielectric constant of about 80. Ice at its
melting point has a dielectric constant of about 3.0 at these frequencies, so there's a huge change in this property in the phase transition from liquid to solid.[4] This fact can lead to useful devices for ice detection.[5]
All this esoteric stuff may be useful, but there's just one thing most people really want to know about snow. Is it really true that no two
snowflakes are alike? At one level, typical snowflakes are alike in their
hexagonal symmetry, as first described in 1611 by the
astronomer,
Johannes Kepler.[6]
René Descartes, the
French philosopher and
mathematician, made drawings of his naked eye observations of snowflakes, which included some twelve-sided flakes, in 1635.[6] The mathematical Descartes reasoned that snowflakes were hexagonal because they needed to arrange themselves efficiently in
clouds. This is likely the first example of
tiling by
efficient causality.
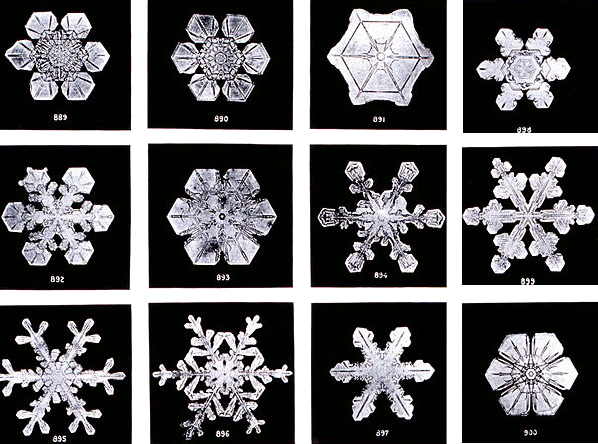
Snowflakes photographed at Jericho, Vermont, by Wilson Alwyn Bentley (1902).
The greatest advance in snowflake science was the invention of the
microscope, which was used by
Robert Hooke to examine everything within reach, including snowflakes. His famous 1665 book,
Micrographia, included many drawings of snowflakes, and these were the first drawings to indicate the complex shapes within shapes in snowflakes. More than two hundred years later, after the invention of
photography,
Wilson Alwyn Bentley, a
Vermont farmer, took numerous
photomicrographs of snowflakes starting in 1885. His photographs documented the fine details inherent in snowflakes and showed how unlikely that two could be the same.
A half century after Bentley started taking his photographs,
deuterium was discovered, as were the
neutron and
isotopes of the
elements. At that point, the idea of two snowflakes being exactly alike became absurd, at least if you were an
atomist. Natural water, as well as ice formed from that water, has a deuterium atom substituting for one of the hydrogen atoms in every 5000th
molecule. The odds of finding a water molecule with
18O substituting for the more common
16O is about one in 500. It doesn't take much
mathematics to conclude that various arrangements of these in the 10
19 water molecules in a snowflake[7] is a very large number.[6] You would think that 10
19 is a large enough number without talking about isotopes, but it's estimated that 10
34 snowflakes have fallen in the history of the
Earth.[7]
Some countries take their snow very seriously, for the obvious reason that they are covered in it for most of the year.
Sweden has issued a set of stamps based on snowflake photographs by
Caltech physicist,
Kenneth G. Libbrecht. Libbrecht, who has a snowflake web site,[6] photographed these particular snowflakes in
Kiruna, Sweden, in an area known as
Lapland (
Santa reindeer territory).
There's a mathematical object known as the
Koch Snowflake. It's a
fractal curve invented by the
Swedish mathematician,
Helge von Koch. What's surprising to people who think that fractals are a modern invention, Koch described this curve in a 1904 paper. It has fractal dimension log 4/log 3, or about 1.26. The snowflake, as shown in the figure, is simply constructed, as follows:[9]
• Draw an equilateral triangle.
• Taking each line segment in turn, divide it into three equal segments.
• Draw an equilateral triangle pointing outwards using the middle segment as the base.
• Remove the base.
• Continue this operation for the next two line segments of the original triangle; and then recursively for all line segments.
There may be applications for such strange objects as the Koch Snowflake. There's a Koch Snowflake Antenna with interesting broadband properties.[10]
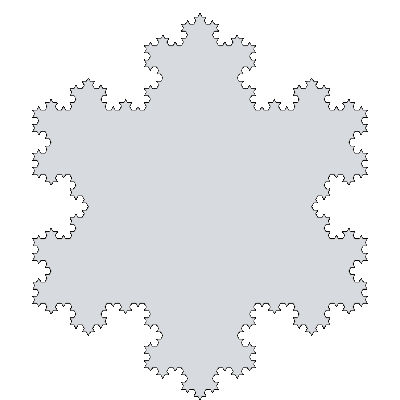
Koch snowflake in its fourth iteration (Image by Wrtlprnft).
![]()
References:
- This blog, "Room Temperature Ice," September 10, 2008.
- K.B. Jinesh and J.W.M.Frenken, "Experimental Evidence for Ice Formation at Room Temperature," Physical Review Letters vol. 101, no. 3 (July 15, 2008) 036101.
- Martin Chaplin, "Anomalous properties of water," London South Bank University, April 6, 2010.
- This huge change occurs only at high frequencies, not at low frequencies. See, for example, Marcus E. Hobbs, Mu Shik Jhon, And Henry Eyring, "The Dielectric Constant Of Liquid Water And Various Forms Of Ice According To Significant Structure Theory," Proc. Natl. Acad. Sci., vol. 56, no. 1 (July 1, 1966), pp. 31-38 .
- Devlin M. Gualtieri, "Aircraft icing sensor," U.S. Patent No. 7,683,791 (Mar 23, 2010)
- Kenneth G. Libbrecht, "SnowCrystals.com," California Institute of Technology.
- John Roach, "No Two Snowflakes the Same" Likely True, Research Reveals," National Geographic News, February 13, 2007.
- Caltech physicist's images featured on Swedish stamps, Whittier Daily News, November 27, 2010.
- Koch Snowflake page on Wikipedia.
- KB7QHC, "Koch Snowflake Antenna," QSL.net.
- Ice page on Wikipedia.
Permanent Link to this article
Linked Keywords: Snow; Northern New Jersey; statistical fluctuation; winter; US southern states; ice; Kurt Vonnegut; Cat's Cradle; ice-nine; ice IX; Kelvin; pascal; MPa; density; water; hydrogen bond; anomalous; dielectric constant; MHz frequencies; melting point; snowflake; hexagonal; symmetry; astronomer; Johannes Kepler; René Descartes; French; philosopher; mathematician; cloud; tiling; efficient causality; Jericho, Vermont; Wilson Alwyn Bentley; microscope; Robert Hooke; Micrographia; photography; photomicrograph; deuterium; neutron; isotope; elements; atomic theory; atomist; molecule; Oxygen-18; 18O; 16O; mathematics; Earth; Sweden; California Institute of Technology; Caltech; physicist; Kenneth G. Libbrecht; Kiruna, Sweden; Lapland; Santa; reindeer; Koch Snowflake; fractal; Swedish; Helge von Koch; Aircraft icing sensor; U.S. Patent No. 7,683,791.