| Tikalon Blog is now in archive mode.
An easily printed and saved version of this article, and a link
to a directory of all articles, can be found below: |
|
This article |
| Directory of all articles |
Graphene Resonators
June 24, 2011
If it wasn't for
quantum mechanics, the
universe would have wound down a long time ago. The essential idea of the quantum, that tiny things, like
electrons in
atoms, remain in a definite
energy state until knocked really hard, is important, since
macroscopic mechanics has one particular problem. A free
oscillator, such a
pendulum, has a lesser and lesser swing over time, and then it stops. A mechanism in a pendulum-based
clock needs to push on the pendulum after each cycle to keep it going.
Friction forces in the pendulum pivot convert some of its motional energy to
heat, so the
harmonic oscillating motion of the pendulum is damped. The equation of motion of a
damped harmonic oscillator is well developed and understood:
x(t) = e-δωot(A cos(ωdt) + A sin(ωdt))
where x(t) is the position at time t, ω
o is the undamped oscillation frequency, δ is the damping coefficient, ω
d is the damped oscillation frequency, and A and B are the initial conditions of motion; viz,
ωd = ωo sqrt(1 - δ2)
A = x(0)
B = (1/ωd)(δωox(0) + [dx/dt]0)
where x(0) is the position at time zero, [dx/dt]
0 is the derivative of position evaluated at time zero, and the damping coefficient is between zero and one.
The plot below shows the
amplitude of a damped harmonic oscillator having an undamped frequency ω
o = 1
rad/sec, a damping coefficient of 0.1, an initial position of zero, and a derivative at zero of one sec
-1. The damping causes a reduction in oscillating frequency to ω
d = 0.995 rad/sec.
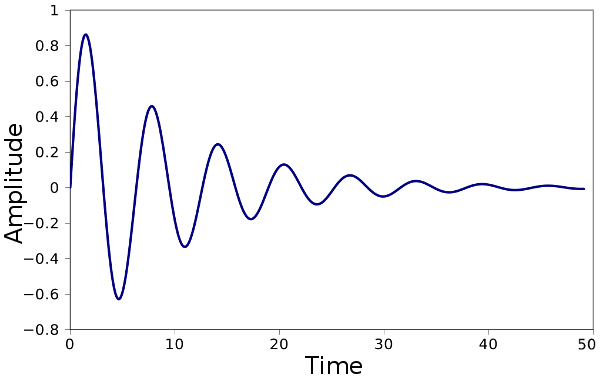
Amplitude of a damped harmonic oscillator. See text for parameters. (Plot via Gnumeric)
As we reduce the size of resonators to
nanoscale dimension, what factors affect the damping? A paper in
Nature Communications lists the following factors:[1]
1. Phonon–phonon interactions that cause thermoelastic damping.
2. Viscous or fluidic damping by the surrounding medium.
3. Material losses caused by the relaxation of bulk and surface defects.
4. Losses from the resonator supporting structure.
A team of researchers from the
Quantum NanoElectronics Group at the
Catalan Institute of Nanotechnology (
Barcelona, Spain), and the
Technische Universität München (
Garching, Germany), found that interesting things happen when the mechanical resonator is shrunk as far as possible. The team fabricated nanoscale resonators of
graphene sheets and
carbon nanotubes by suspending them over grooves in a substrate and clamping the ends.[2-4]
Whereas the damping forces observed in resonators down to a few tens of nanometers in scale are
linear, the team found that resonators of nanoscale graphene and carbon nanotubes show strong
nonlinear damping forces. This non-linearity allowed fabrication of resonators with a high
quality ("Q") factor. They were able to make a graphene resonator with a Q of 100,000, a new record.
Since these resonators have high Q-factor and low mass, they would make excellent force and mass
sensors. The addition of even a small mass, such as an
analyte bonding to a sensitized surface, would cause a large change in the resonance frequency.
References:
- Garrett D. Cole, Ignacio Wilson-Rae, Katharina Werbach, Michael R. Vanner and Markus Aspelmeyer, "Phonon-tunnelling dissipation in mechanical resonators," Nature Communications, vol. 2, article 231, March 8, 2011.
- A. Eichler, J. Moser, J. Chaste, M. Zdrojek, I. Wilson-Rae and A. Bachtold, "Nonlinear damping in mechanical resonators made from carbon nanotubes and graphene," Nature Nanotechnology, Published online May 15, 2011.
- Supplementary Information for Ref. 2.
- Ana de la Osa, "Exotic behavior when mechanical devices reach the nanoscale," Institut Catala de Nanotecnologia Press Release, May 15, 2011
Permanent Link to this article
Linked Keywords: Quantum mechanics; universe; electron; atom; energy level; energy state; macroscopic mechanics; oscillator; pendulum; clock; friction forces; heat; harmonic oscillator; damped harmonic oscillator; amplitude; radian per second; rad/sec; Gnumeric; nanoscale; Nature Communications; phonon; thermoelastic damping; viscosity; fluidic; crystallographic defect; Quantum NanoElectronics Group; Catalan Institute of Nanotechnology; Barcelona, Spain; Technical University Munich; Technische Universität München; Garching bei München; Garching, Germany; graphene sheet; carbon nanotube; linear; nonlinear; Q-factor; quality factor; sensor; analyte.